studioslooki.blogg.se - Recursive formula for geometric sequence

RECURSIVE FORMULA FOR GEOMETRIC SEQUENCE FOR FREE
RECURSIVE FORMULA FOR GEOMETRIC SEQUENCE TRIAL
RECURSIVE FORMULA FOR GEOMETRIC SEQUENCE SERIES

RECURSIVE FORMULA FOR GEOMETRIC SEQUENCE SERIES

Let a n be the n th term of the series and d be the common difference.Īnswer: The recursive formula for this sequence is a n = a n-1 + 5Įxample 3: The 13 th and 14 th terms of the Fibonacci sequence are 144 and 233 respectively. Given that f(0) = 0.Įxample 2: Find the recursive formula for the following arithmetic sequence: 1, 6, 11, 16.

RECURSIVE FORMULA FOR GEOMETRIC SEQUENCE TRIAL

With Cuemath, find solutions in simple and easy steps.īook a Free Trial Class Examples Using Recursive RuleĮxample 1: The recursive formula of a function is, f(x) = 5 f(x-2) + 3, find the value of f(8). Use our free online calculator to solve challenging questions. Let us see the applications of the recursive formulas in the following section. Where a n is the n th term of the sequence.

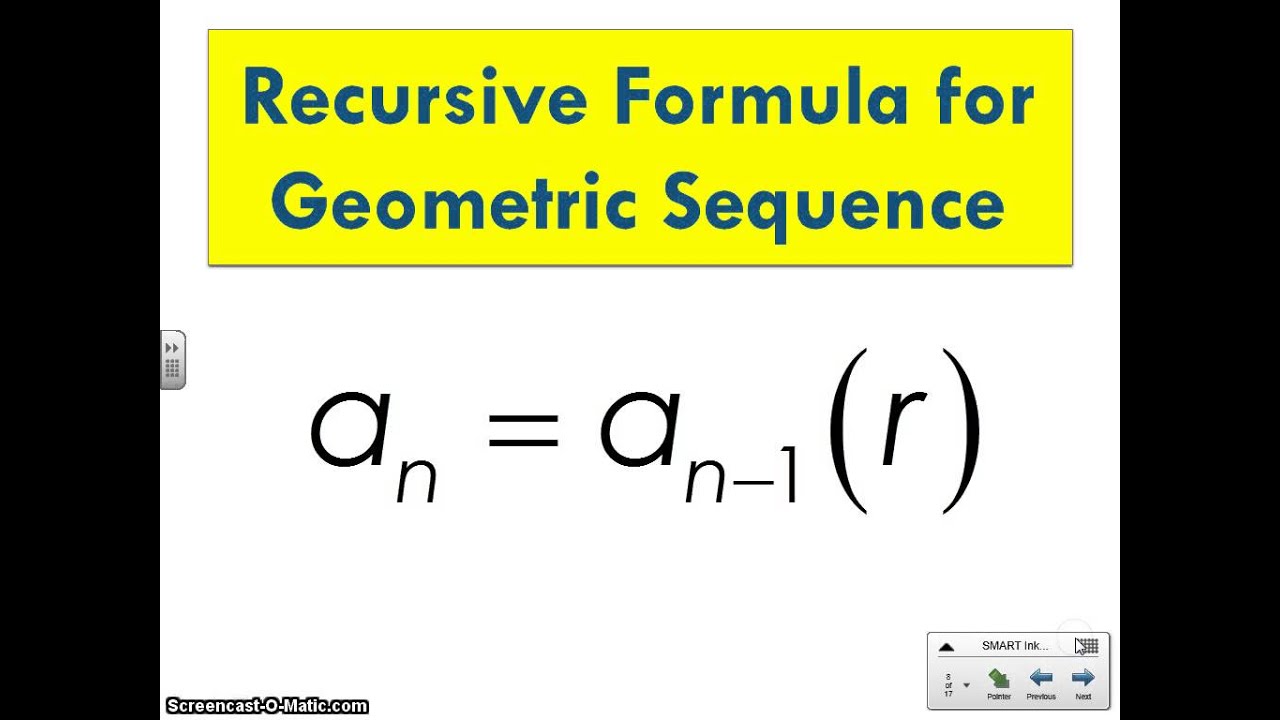
The recursive formula to find the n th term of a Fibonacci sequence is: The recursive formula to find the n th term of a geometric sequence is: The recursive formula to find the n th term of an arithmetic sequence is: Recursive Formula for Arithmetic Sequence The following are the recursive formulas for different kinds of sequences.

The pattern rule to get any term from its previous term.

The recursive formulas define the following parameters: What Are Recursive Formulas?Ī recursive formula refers to a formula that defines each term of a sequence using the preceding term(s). Let us learn the recursive formulas in the following section. + a x-1 h(x-1) where a i ≥ 0 and at least one of the a i > 0 A recursive function h(x) can be written as: where the next term is dependent on one or more known previous term(s). A recursive function is a function that defines each term of a sequence using a previous term that is known, i.e. OpenStax CNX.Before going to learn the recursive formula, let us recall what is a recursive function.

RECURSIVE FORMULA FOR GEOMETRIC SEQUENCE FOR FREE

You can also download for free at For questions regarding this license, please contact If you use this textbook as a bibliographic reference, then you should cite it as follows: This work is licensed under a Creative Commons Attribution 4.0 International License. Glossary common ratio the ratio between any two consecutive terms in a geometric sequence geometric sequence a sequence in which the ratio of a term to a previous term is a constant

In application problems, we sometimes alter the explicit formula slightly to.

An explicit formula for a geometric sequence with common ratio.

As with any recursive formula, the initial term of the sequence must be given.

A recursive formula for a geometric sequence with common ratio.

The terms of a geometric sequence can be found by beginning with the first term and multiplying by the common ratio repeatedly.

The common ratio can be found by dividing any term in the sequence by the previous term.

The constant ratio between two consecutive terms is called the common ratio.

A geometric sequence is a sequence in which the ratio between any two consecutive terms is a constant.

Key Equations recursive formula for n t h Multiplying any term of the sequence by the common ratio 6 generates the subsequent term.Īccess these online resources for additional instruction and practice with geometric sequences. The sequence below is an example of a geometric sequence because each term increases by a constant factor of 6.

Each term of a geometric sequence increases or decreases by a constant factor called the common ratio. The yearly salary values described form a geometric sequence because they change by a constant factor each year. In this section, we will review sequences that grow in this way. When a salary increases by a constant rate each year, the salary grows by a constant factor. His salary will be $26,520 after one year $27,050.40 after two years $27,591.41 after three years and so on.

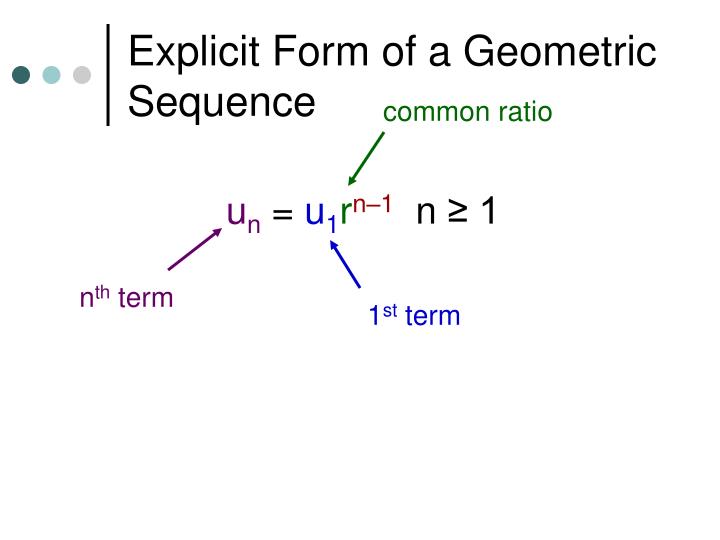
His annual salary in any given year can be found by multiplying his salary from the previous year by 102%. He is promised a 2% cost of living increase each year. Suppose, for example, a recent college graduate finds a position as a sales manager earning an annual salary of $26,000. Many jobs offer an annual cost-of-living increase to keep salaries consistent with inflation. Use an explicit formula for a geometric sequence.

Use a recursive formula for a geometric sequence.

List the terms of a geometric sequence.

Find the common ratio for a geometric sequence.